Where the Sidewalk Ends: What to do on the SAT when you have no idea what to do next

This was one of my favorite books when I was little. And I love reading it to my little girl now. We often talk about what to do when you come to the end of the road and don’t know what to do next.

It comes into play with SAT tutoring as well.

No matter how many SAT and ACT math problems I have answered (around 3000 and counting), I still come across questions I have no idea how to solve. (I’m not sure if this is a testament to my inability to memorize math formulas or a testament to the deviousness of the test writers.)

Many of the kids I tutor are like me: we know enough to get by, but get a little confused when we see those complicated math questions on the SAT and ACT.

1. If you know how to solve it directly, do so.

Of course, when you know how to answer the question, you do so. When you know the formula to use, you immediately apply it and solve for the right answer.

But what do you do when you don’t know what to do next?

2. If you don’t know, start thinking

I spend a lot of my tutoring time in this gray area – what to do when you have no idea what to do next.

Here’s a problem I was working on with one of my students today. It’s from the official SAT Practice Test 1, Section 4, Question 24.

Of course, if you know the freaking circle formula, you could just use it and solve for the left side of the equation. And then, if you had memorized the distance formula, you could use that and solve for the length of the radius.

But what if you didn’t know either one? (And most of my kids don’t know the first and can’t remember the second.) Is there another way to solve for this? What do you do when the sidewalk ends?

What do you know?

I have no idea what the equation of a circle is.* I only know the equation of a line and the Pythagorean theorem and really can’t stuff one more equation into my head.

But, hmmm, I know that all points make a the equation of a line true, and surely that must hold for circles too. So what if I stuff the points into the equation and see which ones equal r^2.

But – argh – I don’t know r either. Hmm.. can I figure out r?

Let me a draw a picture so I can see what I’m looking at.

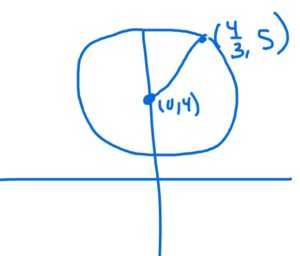
I have a line. And if I knew the awesome distance formula I could use that to solve for the length of the radius. But I don’t know that.

But – we are on a coordinate plane. And I can draw a triangle. And I know the lengths of the legs of the triangle. Yahoo – I can use the Pythagorean theorem to solve for the hypotenuse, which is the radius.

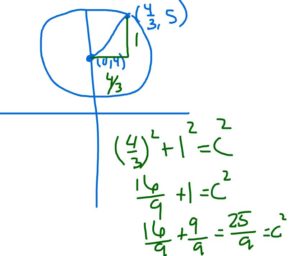
So c=5/3. So I can eliminate answers B and D. Either A or C could be correct – depending on if the circle formula equals r or r^2.

Now I can plug in x and y and see which equation is true.

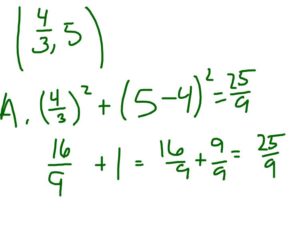
Aha! A works.

And C doesn’t work.

The answer is A.

So think it through.

Leverage what you DO know to figure out what you DON’T know.

Invariably, you’ll encounter questions you don’t know how to solve on the SAT or ACT (and in life.) It’s what you do next that matters.

3. If you really have no idea, choose A

Here’s why A is a better choice than just randomly guessing.

*After reviewing this problem over and over with students, I have now accidentally memorized the equation of a circle: (x-h)^2 + (y-k)^2=r^2